We Can Predict Changes in Consumption by Multiplying the Change in
How changes to the data modify the mean, median, mode, range, and IQR

How measures of central tendency and spread are affected by changes to the data fix
In this section, nosotros want to see what happens to our measures of central tendency and spread when nosotros make changes to our data set.
Specifically the changes made either by changing all the values in the set at one time, or by adding a unmarried data betoken to, or removing a unmarried data point from, the data set.

Hi! I'm krista.
I create online courses to help y'all stone your math grade. Read more.
Irresolute the entire information set
Shifting (addition and subtraction)
What happens to measures of central tendency and spread when we add together a constant value to every value in the data fix? To respond this question, let's pretend nosotros have the data set ???iii,\ 3,\ 7,\ 9,\ 13???, and allow's summate our measures for the gear up.
Hateful: ???(3+three+seven+nine+13)/5=7???
Median: ???7???
Style: ???three???
Range: ???13-3=10???
IQR: ???11-iii=8???
If we add ???half-dozen??? to each information signal in the set, the new set is ???9,\ ix,\ 13,\ fifteen,\ xix???. And our new measures of central tendency and spread are
Mean: ???(9+nine+13+15+nineteen)/5=thirteen???
Median: ???thirteen???
Mode: ???9???
Range: ???nineteen-9=10???
IQR: ???17-9=eight???
What nosotros see is that calculation ???vi??? to the unabridged information set up also adds ???6??? to the mean, median, and mode, simply that the range and IQR stay the same.
And this will always exist truthful. No matter what value we add to the set, the hateful, median, and mode will shift past that amount just the range and the IQR will remain the aforementioned. The same volition be truthful if we subtract an amount from every information point in the set: the mean, median, and mode will shift to the left but the range and IQR volition stay the same.
And so to summarize, whether we add a constant to each data point or subtract a constant from each data point, the mean, median, and way will alter by the aforementioned amount, but the range and IQR will stay the same.
Scaling (multiplication and division)
Let's look at what happens when we multiply our information set by a constant value. Again starting with the set ???three,\ 3,\ 7,\ 9,\ xiii???, the measures are
Mean: ???(3+iii+seven+9+13)/5=7???
Median: ???7???
Fashion: ???iii???
Range: ???13-3=x???
IQR: ???11-3=8???
Allow's multiply the set by ???2???, making the new gear up ???6,\ vi,\ fourteen,\ eighteen,\ 26???. The new measures of fundamental tendency and spread are
Mean: ???(6+6+14+18+26)/five=xiv???
Median: ???xiv???
Mode: ???6???
Range: ???26-6=20???
IQR: ???22-6=16???
What nosotros see is that multiplying the entire data set by ???two??? multiplies all five measures by ???2??? as well. The mean, median, mode, range, and IQR are all doubled when we double the values in the data set.
And this volition always be truthful. No matter what value we multiply by the information set, the mean, median, mode, range, and IQR will all exist multiplied by the same value. The same will be true if we divide every information bespeak in the set past a abiding value: the hateful, median, fashion, range, and IQR will all be divided by the same value.
So to summarize, if we multiply our data set by a constant value or carve up our data prepare by a constant value, then the mean, median, mode, range, and IQR will all exist scaled past the same corporeality.
Adding or removing a information point from the set
Mean
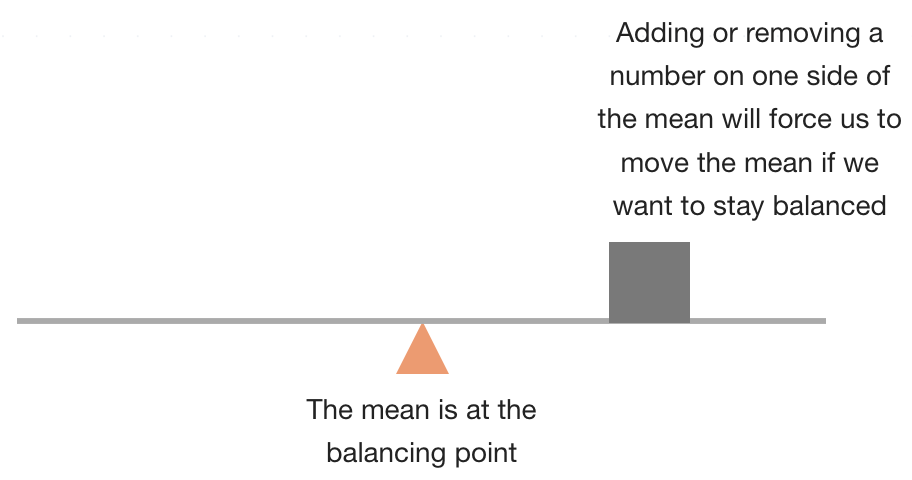
Thinking back to our word about the mean as a balancing point, we desire to realize that adding another data indicate to the data set will naturally event that balancing signal. In fact, adding a data point to the set, or taking 1 away, tin can event the hateful, median, and mode.
If we add a data point that's higher up the mean, or take away a data point that's below the hateful, then the mean will increase. If accept away a data signal that'southward higher up the mean, or add a data indicate that'south below the mean, the mean will subtract.
Median
If we add or remove a data indicate from the set, it can effect the median, merely it may non. In the ready ???1,\ 2,\ iii,\ 4,\ 4,\ six,\ 6???, the median is ???4???. If we take out ???3???, the median of ???1,\ 2,\ 4,\ 4,\ half-dozen,\ 6??? is still ???four???; it's unchanged. But if nosotros take out a ???six???, the median of ???1,\ 2,\ iii,\ iv,\ 4,\ half dozen??? is now ???iii.5???; information technology changes. The aforementioned volition be true for calculation in a new value to the data set up. Depending on the value, the median might modify, or it might not.
Upshot on the mean vs. median
It'due south too of import that we realize that adding or removing an farthermost value from the data fix will impact the mean more the median.
Let's take an like shooting fish in a barrel example, and use the data set up ???1,\ ii,\ 3???. The hateful is ???2??? and the median is ???2???. Let's add a huge value to the data fix, like ???1,000???, then that the new data set is ???ane,\ 2,\ 3,\ ane,000???. The mean of this new data prepare is about ???252???, and the median of the new information set is ???2.v???.
What we see is that adding an extreme value to the data set barely had whatever effect on the median at all: information technology went up from ???2??? to ???2.5???. Only adding the new value had an enormous effect on the mean: information technology shifted the mean from ???2??? upwards to ???252???.
How mean, median, and mode are shifted past changes in the data set


Take the course
Want to learn more about Probability & Statistics? I have a pace-by-stride course for that. :)
The effect of removing one outlier data indicate from the set
Example
Permit'southward say nosotros play a round of golf with three friends, and our scores are the set ???70,\ 71,\ 71,\ 103???. What issue does removing the ???103??? have on the mean and median of the set?
In a set similar this one, nosotros accept a few data points clustered tightly together, and then a data point that is much different than the others. Removing the information point that's far from the cluster effects the mean and median in interesting ways. We can meet that the median of the set is ???71???, and we can calculate that the mean is
???\mu=\frac{70+71+71+103}{four}=\frac{315}{4}\approx79???

No affair what value we add to the set, the mean, median, and way will shift by that corporeality simply the range and the IQR will remain the same.
If we remove the ???103??? from the data ready, the median doesn't change at all because the median of the gear up ???70,\ 71,\ 71??? is still ???71???. But the mean will change significantly. The new mean is
???\mu=\frac{70+71+71}{three}=\frac{212}{3}\approx71???
Which makes sense, because the single data point of ???103??? would tend to skew the data more by bringing upwards the boilerplate. So when it's removed, the hateful drops back down to a value that more accurately reflects nigh of the scores. On the other manus, the ???103??? barely changes the median, which is why the median didn't modify when we removed the ???103???.
A number that has the ability to alter a data set in this manner is called anoutlier; it'south a number on the extreme upper finish or farthermost lower stop of a data set.
Fashion
The mode could also be effected by adding a data point or taking one away. For example, in the set ???ane,\ 2,\ 3,\ 4,\ 4,\ 6,\ vii???, we could add a ???4??? and it wouldn't change the style. We could also take away a ???2???, and information technology wouldn't change the manner. But, if we were to take away a ???4???, the mode of the set would change from ???iv??? to the set having no mode at all.

Get access to the consummate Probability & Statistics course
Source: https://www.kristakingmath.com/blog/effect-on-mean-median-mode-by-changing-the-data
0 Response to "We Can Predict Changes in Consumption by Multiplying the Change in"
Post a Comment